Statistics is a branch of mathematics concerned with gathering, analysing, organising, interpreting and presenting data in different forms. In any situation of a scientific problem, its statistical model is studied to find a solution. Hence, statistics is an essential part of applied mathematics. For statistical data analysis, there are mainly two types of methods: descriptive method and inferential method. Descriptive method analyses data based on the central tendency (mean, median and mode) and dispersion of the data (variance, deviation) from the central point. Inferential statistics depends on the probability theory of the distribution of data which analyses data in a random manner. In this article, we shall briefly discuss the distribution and its types and try to understand in what sense they are useful for analysing data statistically.
What Is a Distribution?
In simple words, statistical distribution is a collection of all possible values that a variable can attain and what is the frequency of that value. Distribution in probability usually means probability distribution because we consider the chances of a particular event. Distributions can be discrete or continuous depending on the nature of a random variable.
Types of Distributions
Now, let us briefly discuss the common types of distribution we frequently use.
- Bernoulli distribution
- Binomial distribution
- Uniform distribution
- Normal distribution
- Exponential distribution
Bernoulli Distribution
A Bernoulli event is such that the probability of occurrence of that event has only two discrete values, namely success (1) and failure (0) in a single trial. If p is the probability of happening that event, then 1 – p will be the probability of not happening of that event. The probability distribution of a Bernoulli event is called the Bernoulli distribution. Each event in a Bernoulli distribution has a pair of probabilities.
For X is a random variable with a Bernoulli distribution
P(X = 1) = p and P(X = 0) = 1 – p = q
The probability mass function f of this distribution, for possible outcome, k is given by
f(k, p) = p when k = 1(case of success) and f(k, p) = q = 1 – p when k = 0 (case of failure)
We can also express f as
f(k, p) = pk (1 – p)1 – k where k ∈ {0, 1}
Binomial Distribution
Bernoulli distribution is a special case of the binomial distribution. The binomial distribution is a probability distribution of n repeated independent Bernoulli trials. Here, independent refers to the fact that each trial does not affect another, whereas repeated in the sense that conditions for each Bernoulli trial are the same.
The binomial distribution formula is given by –
P(x : n, p) = nCxpx (1 – p)n – x
Where n is the number of repeated independent trials
x = 0, 1, 2, 3, 4, … the number of successes
p = probability of getting success while performing a random experiment.
Uniform Distribution
Uniform distribution is also known as rectangular distribution. The basis of this distribution is the possibility that getting all the outcomes is equally likely. The probability distribution function of a continuous uniform distributiondepends on the two parameters, a and b, where a is the minimum value, and b is the maximum value of the distribution.
f(x) = 1/(b – a) for a ≤ x ≤ b and f(x) = 0 when x ∉ [a, b]
The graph of this type of distribution looks like a rectangle, called rectangular distribution.
Normal Distribution
It is a continuous probability distribution of real-valued random variables, whose mean, median, and mode coincide. The probability distribution function is given by
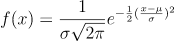
Where μ is the mean, median or mode, σ is the standard deviation, and σ2 is the variance of the distribution.
Normal distributions are the most often used distribution function to represent common problems statistically. The probability distribution curve of a normal distribution is bell-shaped and symmetric about the line x = μ, and the total area under the curve is 1.
The standard normal distribution is a distribution with a mean of zero and a standard deviation of 1. That is, μ = 0 and σ = 1. Then, the probability distribution function will be:
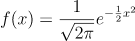
Also, read about the confidence level, which is based on the standard normal distribution.
Exponential Distribution
It is a type of probability distribution which depends on the time between the events. It is a distribution which depicts a process which occurs independently at a constant average rate.
Its distribution function is given by:
f(x) = {λe –λx: x ≥ 0} otherwise f(x) = 0 for x < 0
Where λ is the rate parameter.
Some properties of the exponential distribution function are:
- Mean, or the expected value of the exponential distribution, is determined by: E[x] = 1/λ
- The variance of the random variable x is given by: Var[x] = 1/λ2
- The standard deviation is equal to the mean of the distribution.